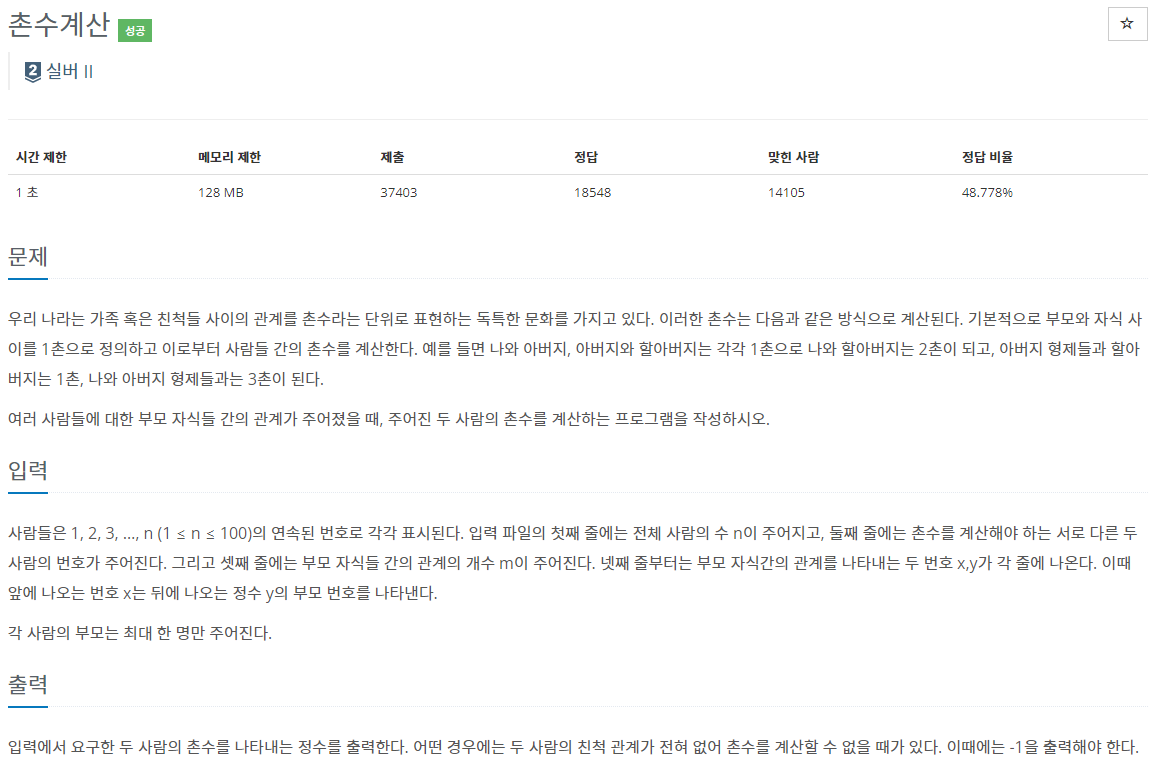
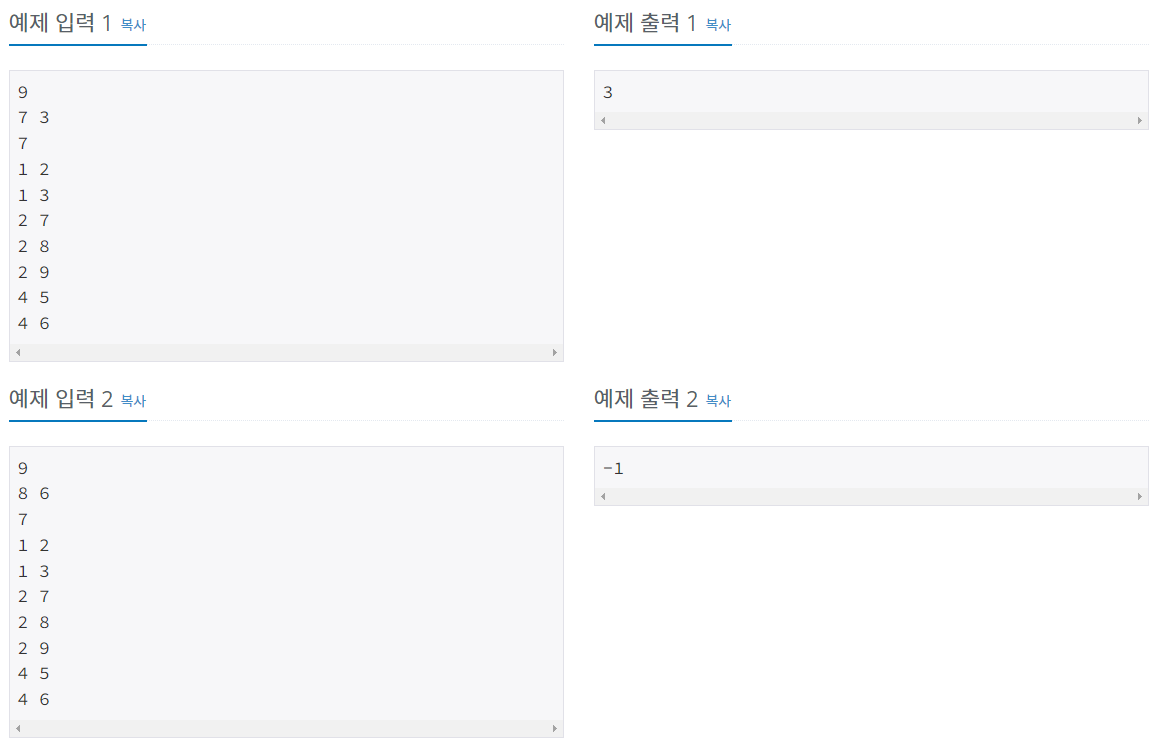
from collections import deque
# 전체 사람 수(n)
n = int(input())
# 촌수를 계산해야 하는 두 사람
target_a, target_b = map(int,input().split())
# 부모 자식들 간의 관계의 개수
m = int(input())
# 전체 사람의 부자 관계를 나타내는 2차원 리스트
graph = [[] for _ in range(n+1)]
# 확인 여부 확인용 리스트
visited = [False] * (n+1)
# 친척 관계를 저장하는 리스트
relation = [0]*(n+1)
relation[target_a] = 0
for _ in range(m):
x, y = map(int,input().split())
graph[x].append(y)
graph[y].append(x)
def bfs(graph,v,visited):
visited[v] = True
queue = deque([v])
while queue:
popped = queue.popleft()
for i in graph[popped]:
if not visited[i]:
visited[i] = True
queue.append(i)
relation[i] = relation[popped]+1
bfs(graph,target_a,visited)
if relation[target_b] == 0:
print(-1)
else:
print(relation[target_b])이번 문제는 여러가지 부모관계가 주어진 상황에서, 특정한 사람(정점)에서 다른 사람(정점)까지 몇 촌 관계(거리)에 있는지 확인하는 문제로, 굉장히 흥미로운 주제의 문제였다.
for _ in range(m):
x, y = map(int,input().split())
graph[x].append(y)
graph[y].append(x)먼저 부모관계는 두 정점이 서로 가지고 있는 양방향 관계이기 때문에 양방향 그래프의 형식으로 저장해주었다.
def bfs(graph,v,visited):
visited[v] = True
queue = deque([v])
while queue:
popped = queue.popleft()
for i in graph[popped]:
if not visited[i]:
visited[i] = True
queue.append(i)
relation[i] = relation[popped]+1또한 특정 정점에서 다른 정점까지의 거리를 기록해야 하기 때문에 BFS 알고리즘을 사용하였다 !
마지막으로 특정 사람을 기준으로 다른 사람들의 친척 관계를 저장하기 위한 배열(relation)을 사용함으로써 최종적인 답을 얻을 수 있었다.
BFS 알고리즘을 양방향 그래프의 방식으로 풀어볼 수 있었던 흥미로운 문제였다 :)
'Algorithm 💡 > BFS' 카테고리의 다른 글
| [백준 2583번] 영역 구하기 (0) | 2023.04.06 |
|---|---|
| [백준 2468번] 안전 영역 (0) | 2023.02.15 |
| [백준 10026번] 적록색약 (0) | 2023.02.14 |
| [백준 7576번] 토마토 (0) | 2023.02.14 |
| [백준 2178번] 미로 탐색 (0) | 2023.02.12 |



